
↑ # Clips = 2
I am enthusiastic about building binder clip sculptures. I made up the name oriclip, which is inspired by my older habit origami, which stands for ori “fold” and kami “paper”.
(In some places, UK and its friends I suppose, binder clips are called foldover clips or foldback clips, which sort-of justifies the prefix ori.)
Fast forward to

↑ # Clips = 2

↑ # Clips = 2

↑ # Clips = 2

↑ # Clips = 6
↑ Base = triangular antiprism
↑ Symmetry = triangular antiprism’s rotations

↑ # Clips = 6
↑ Base = six-piece burr
↑ Symmetry = triangular antiprism’s rotations

↑ # Clips = 6
↑ Base = six-piece burr
↑ Symmetry = tetrahedron’s rotations
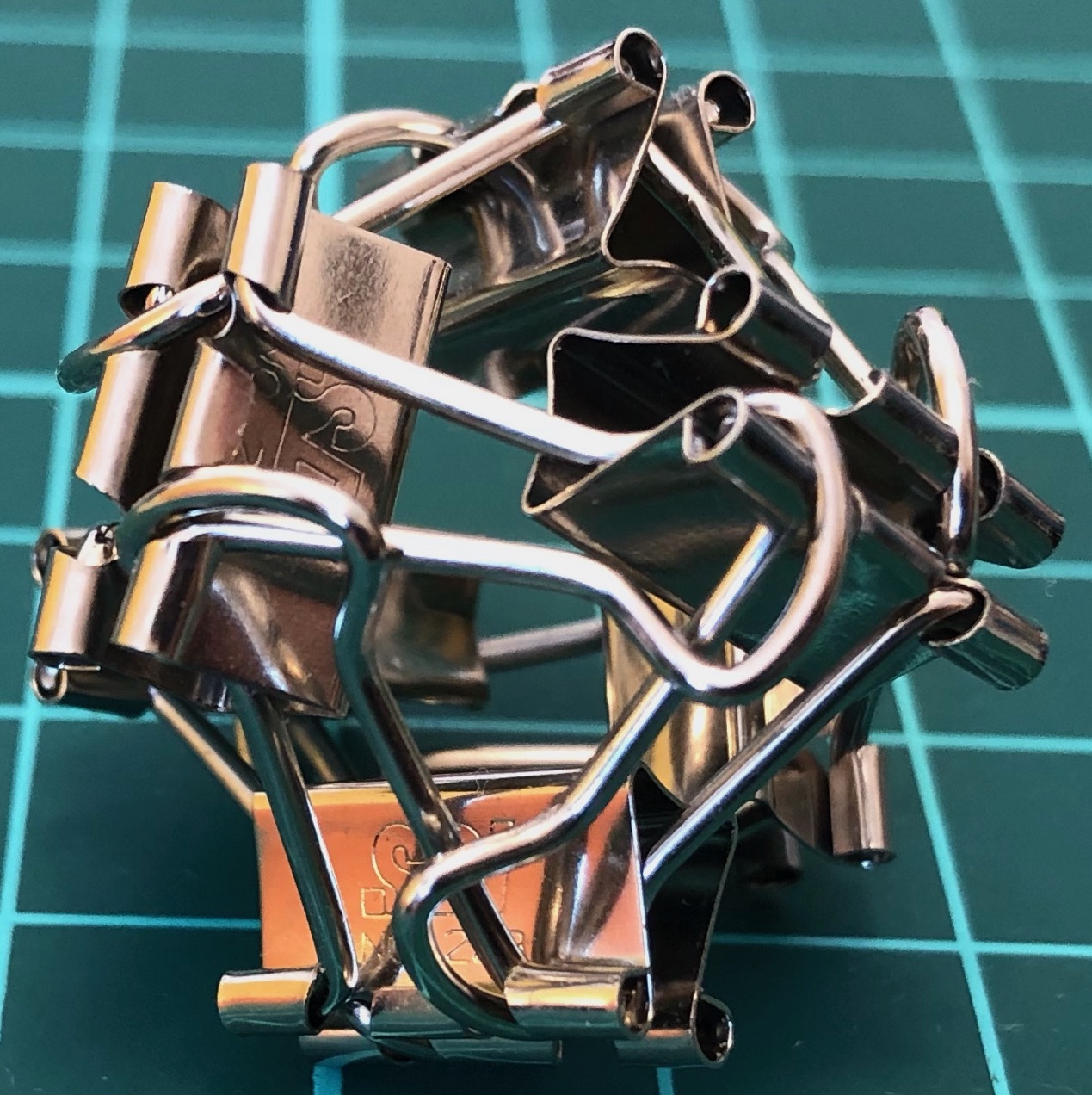
↑ # Clips = 6
↑ Base = octahedron
↑ Symmetry = tetrahedron’s rotations
↑ Video instruction = https://youtu.be/XCLxfR3sDGM

↑ # Clips = 6
↑ Base = octahedron
↑ Symmetry = tetrahedron’s rotations

↑ # Clips = 6
↑ Base = three-piece burr
↑ Symmetry = pyritohedron’s rotations and reflections
↑ Video instruction = https://youtu.be/8F8225Ve_RE
↑ Looks like = Czech hedgehog

↑ # Clips = 6
↑ Base = six-piece burr
↑ Symmetry = pyritohedron’s rotations and reflections
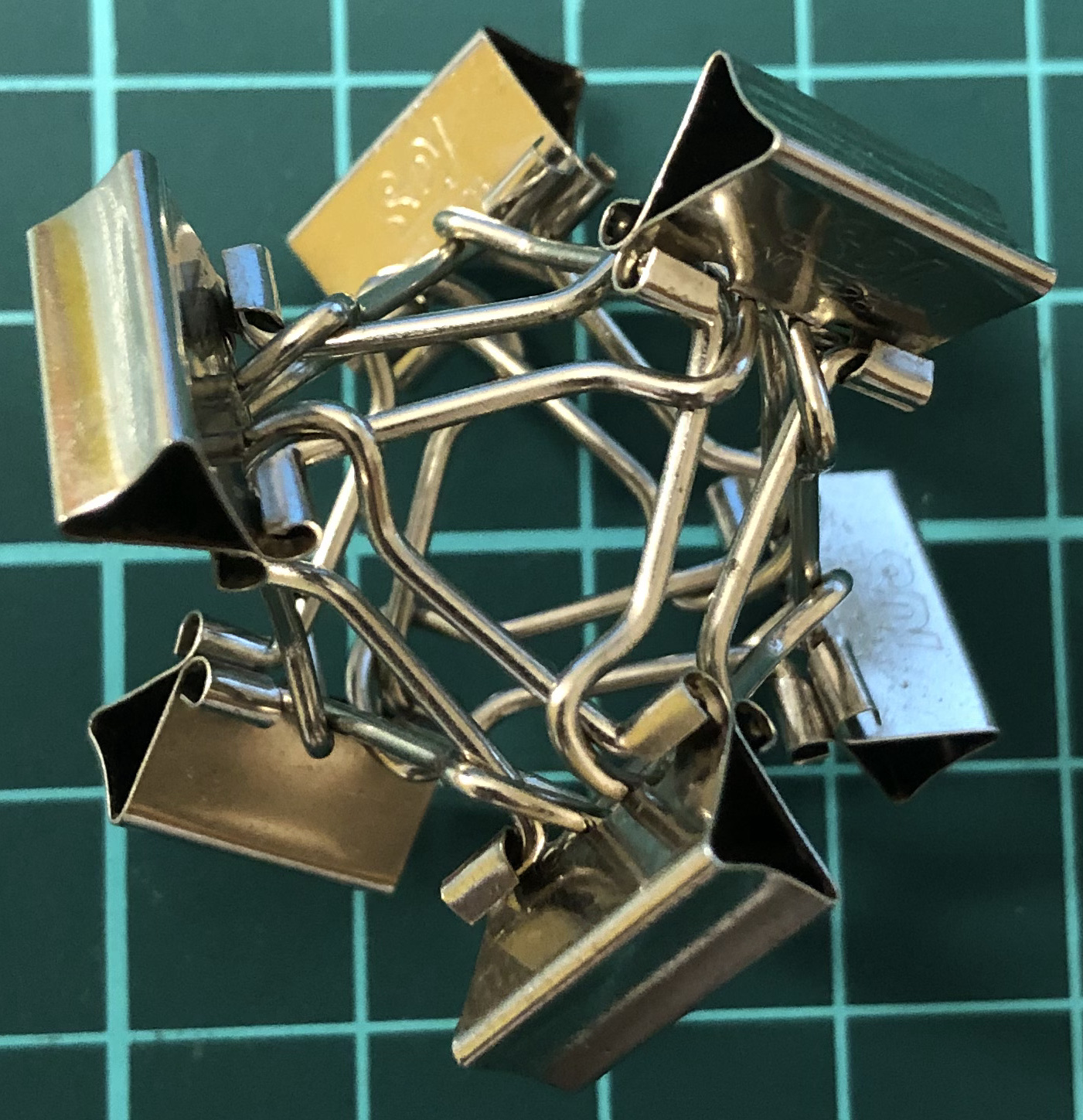
↑ # Clips = 6
↑ Base = octahedron
↑ Symmetry = pyritohedron’s rotations and reflections
 ↑ # Clips = 12
↑ # Clips = 12
↑ Base = octahedron
↑ Symmetry = pyritohedron’s rotations and reflections

↑ # Clips = 12
↑ Base = cuboctahedron
One clip = one vertex. One handle = one edge.

↑ # Clips = 12
↑ Base = cuboctahedron
↑ Symmetry = cube’s rotations
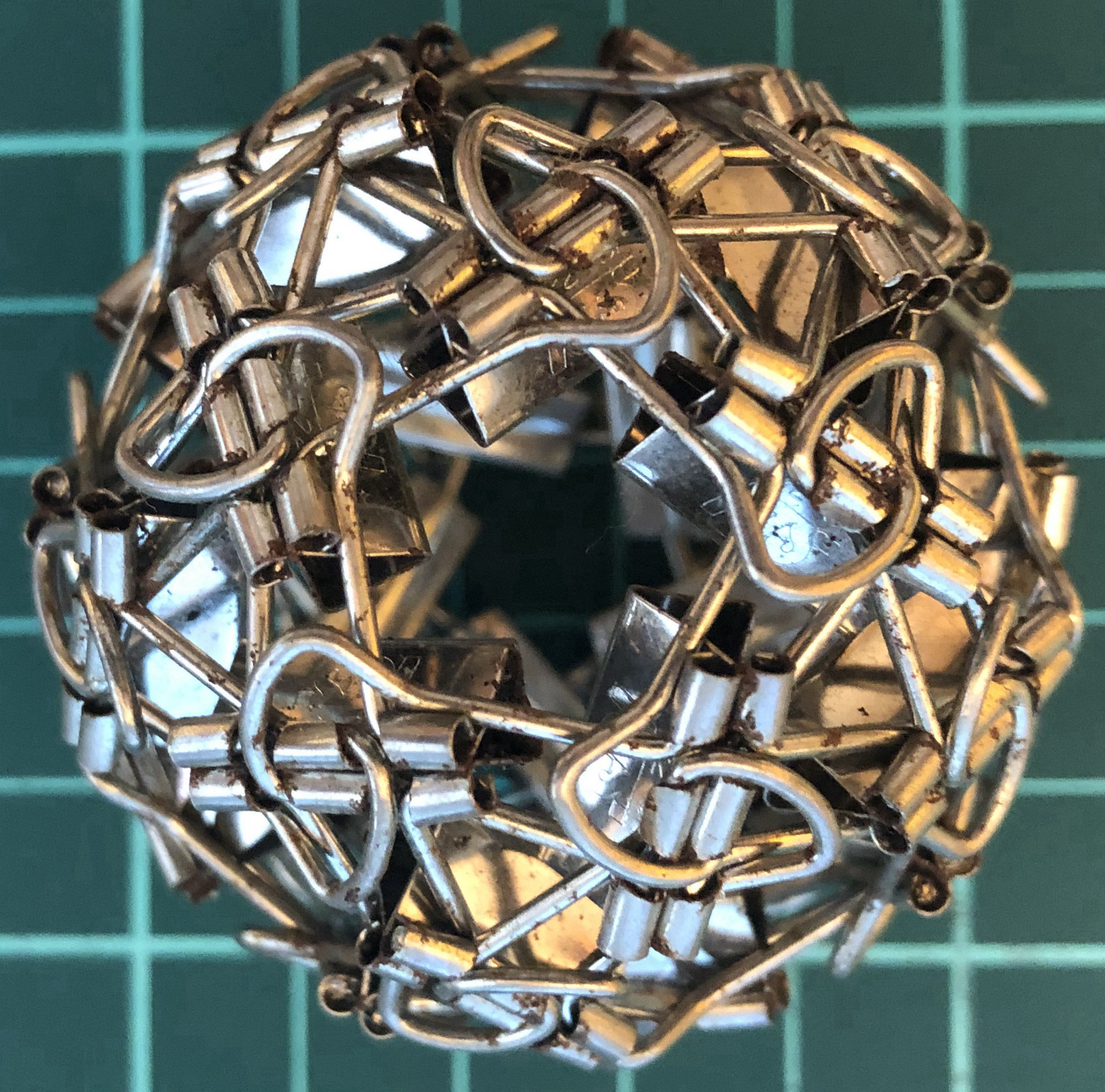
↑ # Clips = 30
↑ Base = icosidodecahedron
↑ Symmetry = dodecahedron’s rotations
One clip = one edge.

↑ # Clips = 12
↑ Base = octahedron
↑ Symmetry = pyritohedron’s rotations and reflections
↑ Video instruction = https://youtu.be/aXINnqdEPB8
↑ Looks like = Ramiel in Evangelion

↑ # Clips = 24
↑ Base = cuboctahedron
↑ Symmetry = cube’s rotations
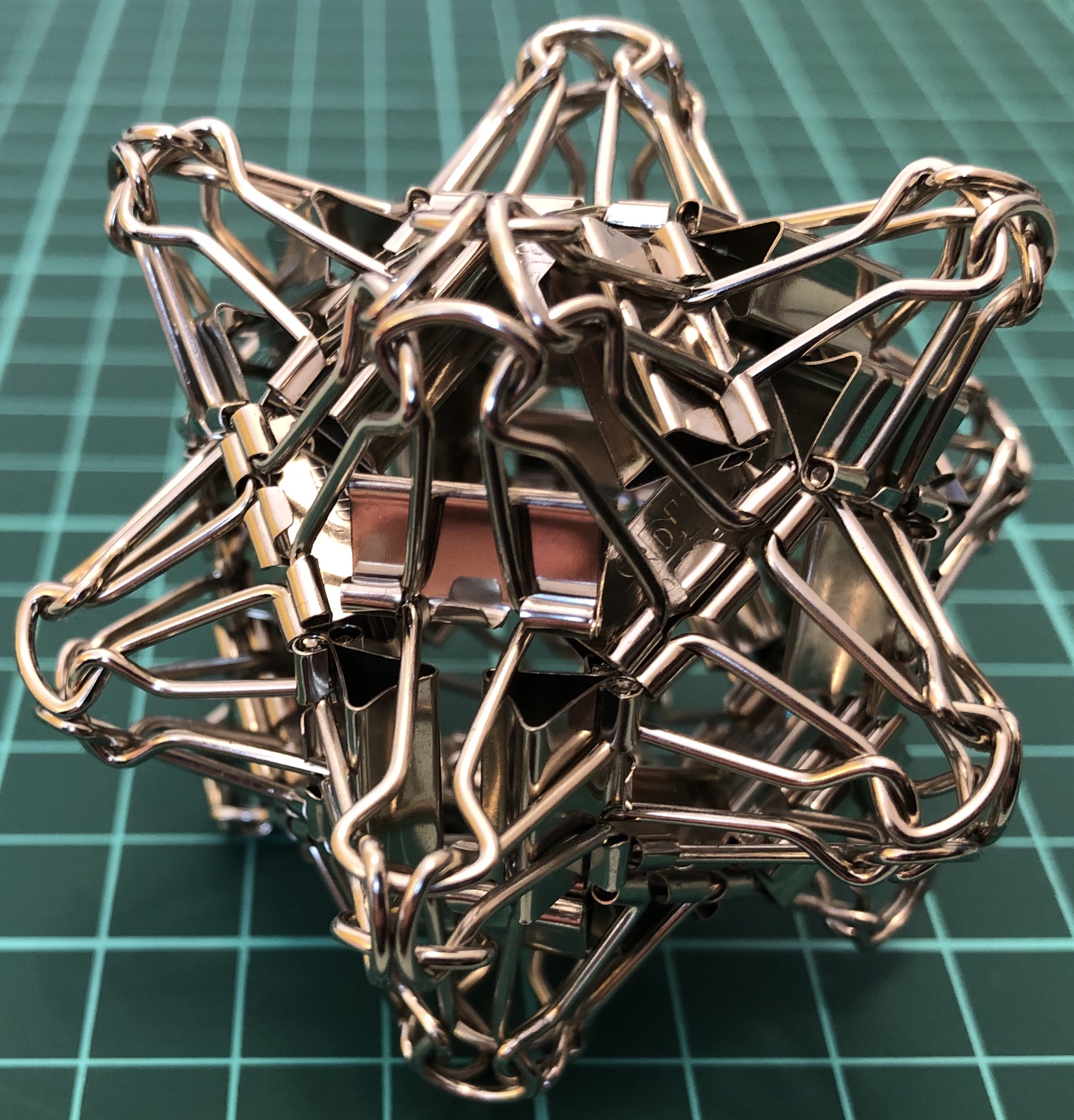
↑ # Clips = 36
↑ Base = tetrakis hexahedron
↑ Symmetry = pyritohedron’s rotations and reflections

↑ # Clips = 48
↑ Base = rhombicuboctahedron
↑ Symmetry = cube’s rotations

↑ # Clips = 60
↑ Base = icosidodecahedron
↑ Symmetry = dodecahedron’s rotations

↑ # Clips = (12 per vertex) x (8 vertices) = 96
↑ Local base = octahedron
↑ Global base = cube
↑ Symmetry = cube’s rotations

↑ # Clips = (24 per vertex) x (4 vertices) = 96
↑ Local base = cuboctahedron
↑ Global base = tetrahedron
↑ Symmetry = tetrahedron’s rotations

↑ # Clips = (24 per vertex) x (8 vertices) = 144
↑ Local base = cuboctahedron
↑ Global base = octahedron
↑ Symmetry = cube’s rotations
Four clips = one Η-vertex = one vertex.

↑ # Clips = (4 per face) x (6 faces) = 24
↑ Base = octahedron
↑ Vertex config = 3.3.3.3
↑ Symmetry = pyritohedron’s rotations and reflections
↑ Video instruction = https://youtu.be/Crru2VOmpL4

↑ # Clips = (4 per vertex) x (12 vertices) = 48
↑ Base = rhombic dodecahedron
↑ Face config = 3.4.3.4
↑ Symmetry = cube’s rotations
 ↑ # Clips = (4 per face) x (30 vertices) = 120
↑ # Clips = (4 per face) x (30 vertices) = 120
↑ Base = icosidodecahedron
↑ Symmetry = dodecahedron’s rotations

↑ # Clips = 120
↑ Base = application of the loft operation upon a cube
↑ Symmetry = cube’s rotations

↑ # Clips = 24
↑ Base = tetrahedron
↑ Vertex config = 3.3.3
↑ Symmetry = tetrahedron’s rotations

↑ # Clips = 48
↑ Base = octahedron
↑ Vertex config = 3.3.3.3
↑ Symmetry = pyritohedron’s rotations and reflections

↑ # Clips = 48
↑ Base = cube
↑ Vertex config = 4.4.4
↑ Symmetry = tetrahedron’s rotations
This “H” is the Latin Ech because it is used as an edge unit. The other “Η” are Greek Eta because they are used as vertex unit.

↑ # Clips = 48
↑ Base = octahedron
↑ Vertex config = 3.3.3.3
↑ Symmetry = cube’s rotations
This “H” is the Latin Ech because it is used as an edge unit. The other “Η” are Greek Eta because they are used as vertex unit.

↑ # Clips = 120
↑ Base = dodecahedron
↑ Vertex config = 5.5.5
↑ Symmetry = dodecahedron’s rotations
Three clips = one Φ-vertex = one vertex.
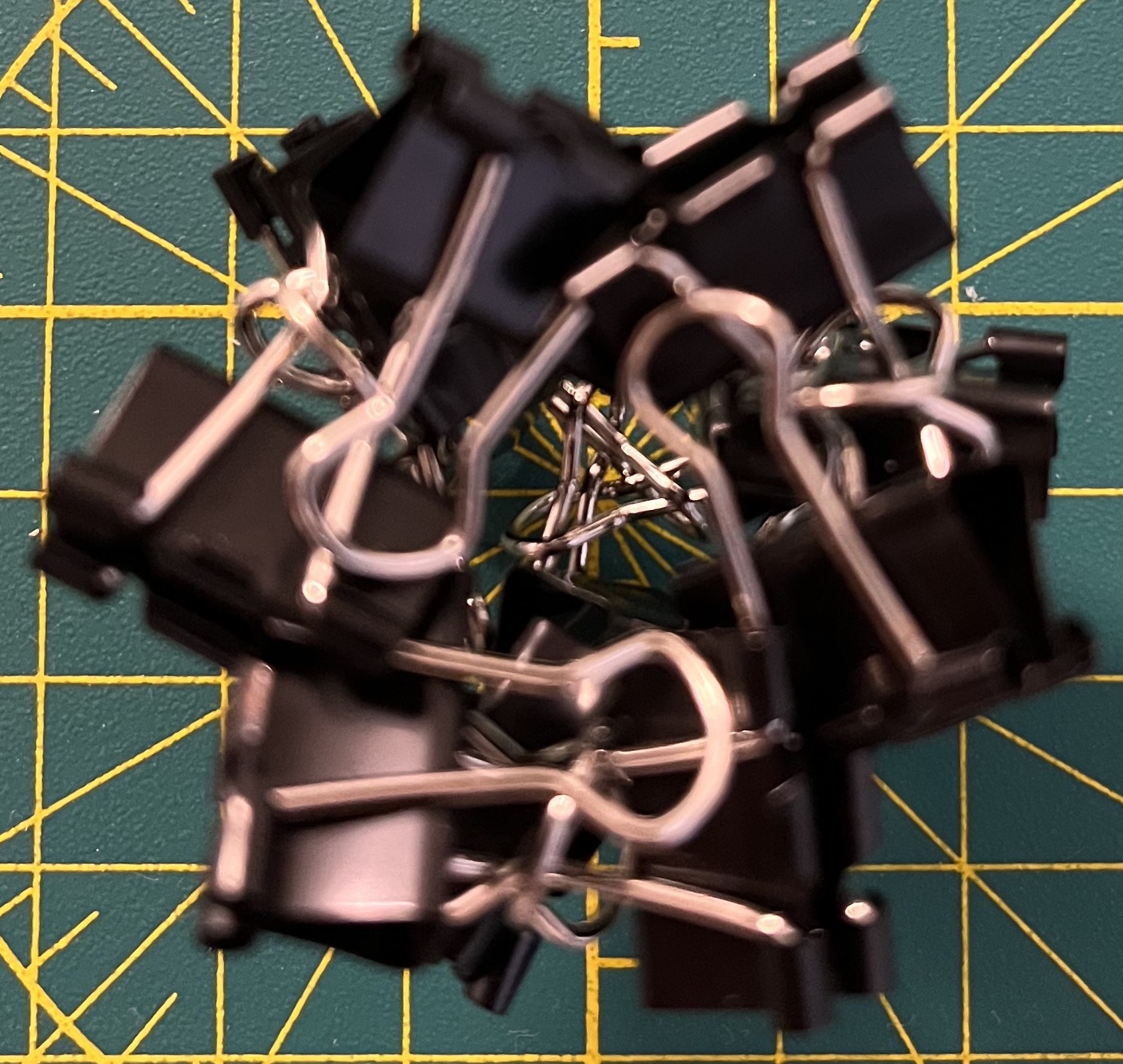
↑ # Clips = 12
↑ Base = tetrahedron
↑ Vertex config = 3.3.3
↑ Symmetry = tetrahedron’s rotations
↑ Dual = itself

↑ # Clips = 24
↑ Base = cube
↑ Vertex config = 4.4.4
↑ Symmetry = cube’s rotations
↑ Dual = Φ24-O

↑ # Clips = 24
↑ Base = octahedron
↑ Vertex config = 3.3.3.3
↑ Symmetry = cube’s rotations
↑ Dual = Φ24-C

↑ # Clips = 60
↑ Vertex config = 3.3.3.3.3
↑ Base = icosahedron
↑ Symmetry = dodecahedron’s rotations

↑ # Clips = 60
↑ Vertex config = 5.5.5
↑ Base = dodecahedron
↑ Symmetry = dodecahedron’s rotations
Three clips = one Ψ-vertex = one vertex.

↑ # Clips = 12
↑ Base = tetrahedron
↑ Vertex config = 3.3.3
↑ Symmetry = tetrahedron’s rotations
↑ Dual = itself

↑ # Clips = 24
↑ Base = cube
↑ Vertex config = 4.4.4
↑ Symmetry = cube’s rotations
↑ Dual = Ψ24-O

↑ # Clips = 24
↑ Base = octahedron
↑ Vertex config = 3.3.3.3
↑ Symmetry = cube’s rotations
↑ Dual = Ψ24-C
Three clips = one Δ-vertex = one vertex.

↑ # Clips = 60
↑ Vertex config = 5.5.5
↑ Base = dodecahedron
↑ Symmetry = dodecahedron’s rotations

↑ # Clips = 180
↑ Vertex config = 5.6.6
↑ Base = truncated icosahedron
↑ Symmetry = dodecahedron’s rotations
Three clips = one Y-edge = one edge.

↑ # Clips = 24
↑ Base = tetrahedron
↑ Vertex config = 3.3.3
↑ Symmetry = tetrahedron’s rotations
Two clips = one X-edge = one edge.

↑ # Clips = (2 per edge) x (6 edges) = 12
↑ Base = tetrahedron
↑ Vertex config = 3.3.3
↑ Symmetry = tetrahedron’s rotations
↑ Dual = itself
↑ Video Instruction = https://youtu.be/0hu1LEuSWS4
↑ Looks like = Roman sueface
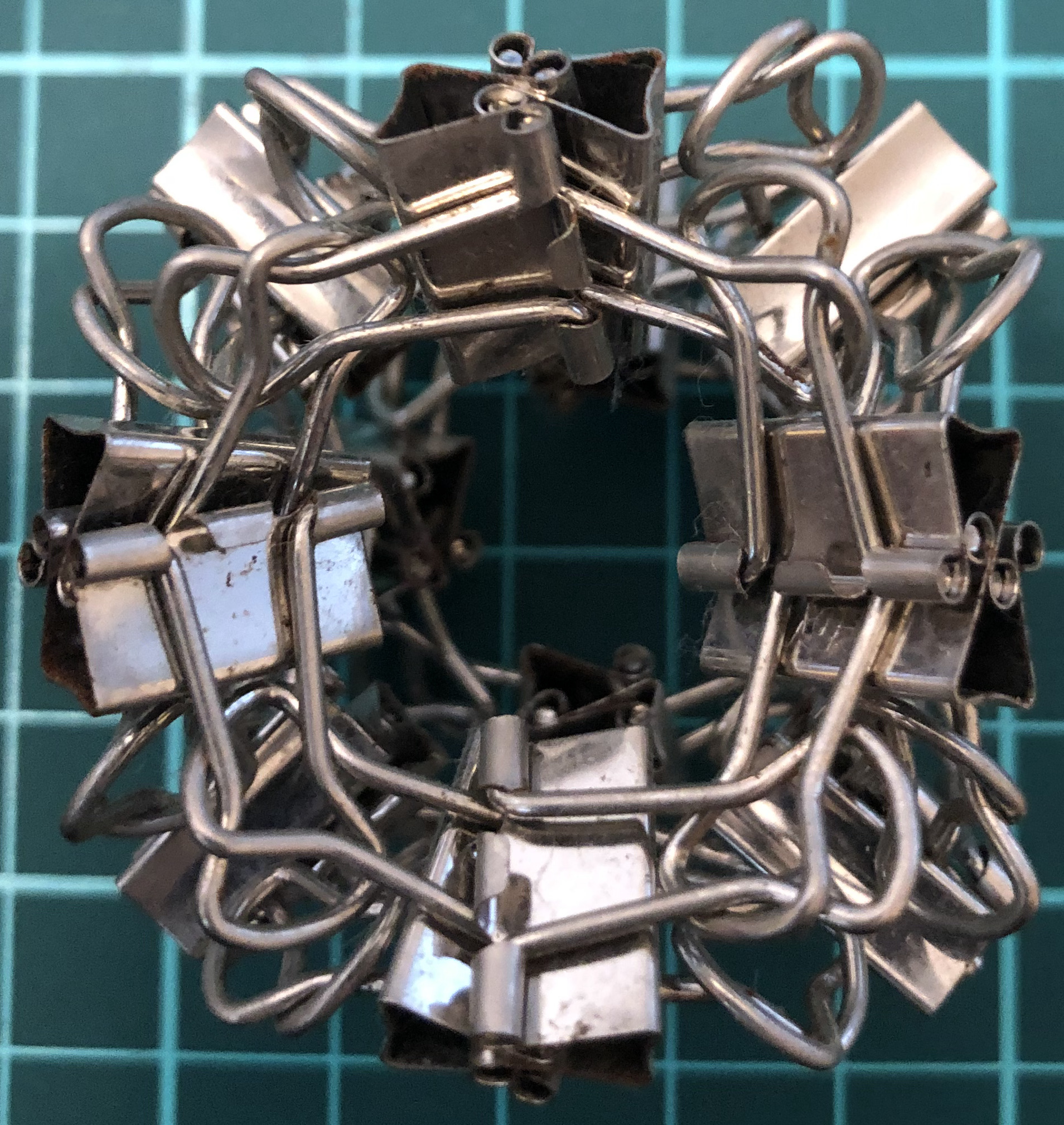
↑ # Clips = 24
↑ base = cube
↑ Vertex config = 4.4.4
↑ Symmetry = cube’s rotations
↑ dual = X24-O

↑ # Clips = 24
↑ base = octahedron
↑ Vertex config = 3.3.3.3
↑ Symmetry = cube’s rotations
↑ dual = X24-C

↑ # Clips = 60
↑ Vertex config = 5.5.5
↑ Base = dodecahedron
↑ Symmetry = dodecahedron’s rotations
↑ dual = X60-I

↑ # Clips = 60
↑ Vertex config = 3.3.3.3.3
↑ Base = icosahedron
↑ Symmetry = dodecahedron’s rotations
↑ dual = X60-D

↑ # Clips = 120
↑ Vertex config = 5.5.5
↑ Base = dodecahedron
↑ Symmetry = dodecahedron’s rotations
Two clips = one L-edge = one edge.
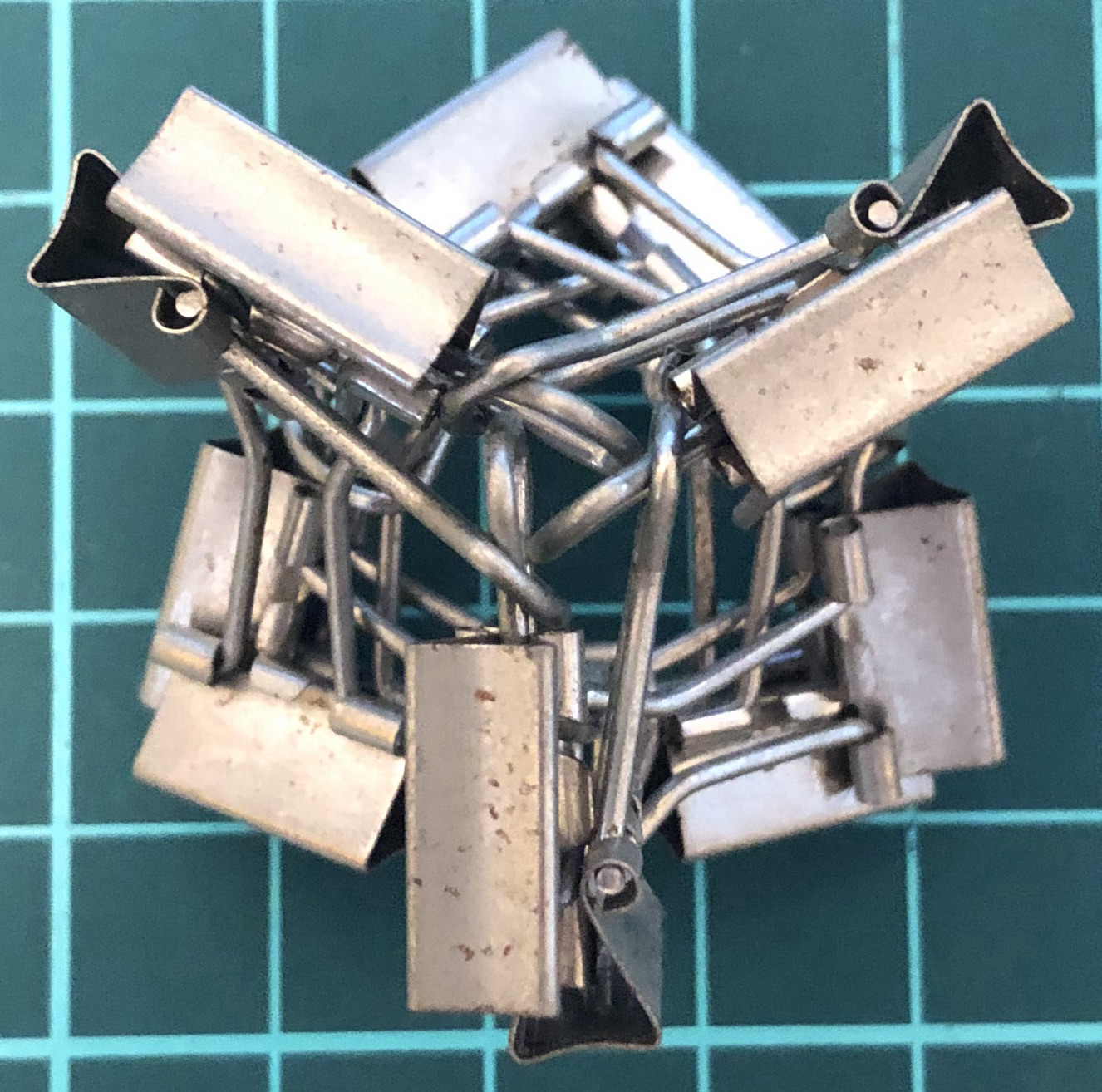
↑ # Clips = 12
↑ Base = tetrahedron
↑ Vertex config = 3.3.3
↑ Symmetry = tetrahedron’s rotations
↑ Dual = itself
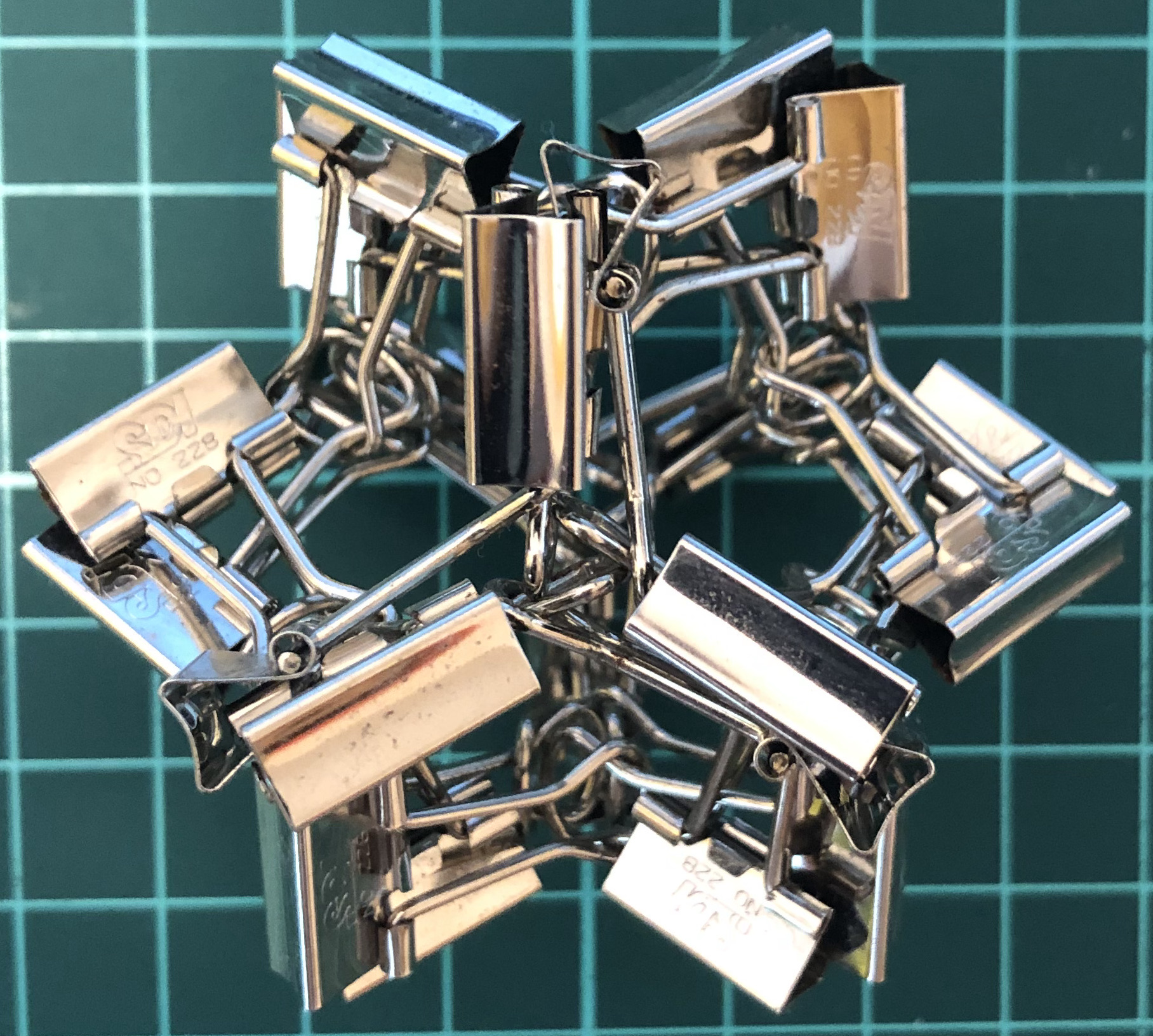
↑ # Clips = 24
↑ Base = cube
↑ Vertex config = 4.4.4
↑ Symmetry = cube’s rotations
↑ Dual = L24-O

↑ # Clips = 24
↑ Base = octahedron
↑ Vertex config = 3.3.3.3
↑ Symmetry = cube’s rotations
↑ Dual = L24-C
↑ Video instruction = https://youtu.be/rpFVjyZ3XF8
↑ Looks like = gyroscope frame

↑ # Clips = 60
↑ Base = dodecahedron
↑ Vertex config = 5.5.5
↑ Symmetry = dodecahedron’s rotations
↑ Dual = L60-I

↑ # Clips = 60
↑ Base = icosahedron
↑ Vertex config = 3.3.3.3.3
↑ Symmetry = dodecahedron’s rotations
↑ Dual = L60-D

↑ # Clips = 36
↑ Base = truncated tetrahedron
↑ Vertex config = 3.6.6
↑ Symmetry = tetrahedron’s rotations

↑ # Clips = 48
↑ Base = cuboctahedron
↑ Vertex config = 3.4.3.4
↑ Symmetry = cube’s rotations

↑ # Clips = 180
↑ Base = truncated icosahedron
↑ Vertex config = 5.6.6
↑ Symmetry = dodecahedron’s rotations
Two clips = one I-edge = one edge.

↑ # Clips = 12
↑ Base = tetrahedron
↑ Vertex config = 3.3.3
↑ Symmetry = tetrahedron’s rotations
↑ Dual = itself

↑ # Clips = 24
↑ base = cube
↑ Vertex config = 4.4.4
↑ Symmetry = cube’s rotations
↑ dual = I24-O

↑ # Clips = 24
↑ Base = octahedron
↑ Vertex config = 3.3.3.3
↑ Symmetry = cube’s rotations
↑ Dual = I24-C

↑ # Clips = 30
↑ Vertex config = 5.5.5
↑ Base = dodecahedron
↑ Symmetry = dodecahedron’s rotations
↑ Dual = I60-I

↑ # Clips = 30
↑ Base = icosahedron
↑ Vertex config = 3.3.3.3.3
↑ Symmetry = dodecahedron’s rotations
↑ dual = I60-D

↑ # Clips = 24
↑ Base = tetrahedron
↑ Vertex config = 3.3.3
↑ Symmetry = tetrahedron’s rotations
↑ Dual = itself

↑ # Clips = 36
↑ Base = truncated tetrahedron
↑ Vertex config = 3.6.6
↑ Symmetry = tetrahedron’s rotations
↑ Dual = I36-kT

↑ # Clips = 48
↑ Base = cuboctahedron
↑ Vertex config = 3.4.3.4
↑ Symmetry = cube’s rotations
↑ Dual = I48-jC

↑ # Clips = 72
↑ Base = truncated cube
↑ Vertex config = 3.8.8
↑ Symmetry = cube’s rotations
↑ (Dual = triakis octahedron)

↑ # Clips = 72
↑ Base = truncated octahedron
↑ Vertex config = 4.6.6
↑ Symmetry = cube’s rotations
↑ I72-kC

↑ # Clips = 96
↑ Base = rhombicuboctahedron
↑ Vertex config = 3.4.4.4
↑ Symmetry = cube’s rotations
↑ I96-jjC

↑ # Clips = 120
↑ Base = snub cube
↑ Vertex config = 3.3.3.3.4
↑ Symmetry = cube’s rotations
↑ (Dual = pentagonal icositetrahedron)

↑ # Clips = 120
↑ Base = icosidodecahedron
↑ Vertex config = 3.5.3.5
↑ Symmetry = dodecahedron’s rotations
↑ Dual = I120-jD

↑ # Clips = 180
↑ Base = truncated icosahedron
↑ Vertex config = 5.5.6
↑ Symmetry = dodecahedron’s rotations
↑ Dual = I180-kD

↑ # Clips = 240
↑ Base = rhombicosidodecahedron
↑ Vertex config = 3.4.5.4
↑ Symmetry = dodecahedron’s rotations

↑ # Clips = 300
↑ Base = snub dodecahedron
↑ Face config = 3.3.3.3.5
↑ Symmetry = dodecahedron’s rotations
↑ (Dual = pentagonal hexecontahedron)

↑ # Clips = 36
↑ Face config = 3.6.6
↑ Base = triakis tetrahedron
↑ Symmetry = tetrahedron’s rotations
↑ Dual = I36-tT

↑ # Clips = 48
↑ Base = rhombic dodecahedron
↑ Face config = 3.4.3.4
↑ Symmetry = cube’s rotations
↑ Dual = I48-aC

↑ # Clips = 72
↑ Base = tetrakis hexahedron
↑ Face config = 4.6.6
↑ Symmetry = cube’s rotations
↑ Dual = I72-tO
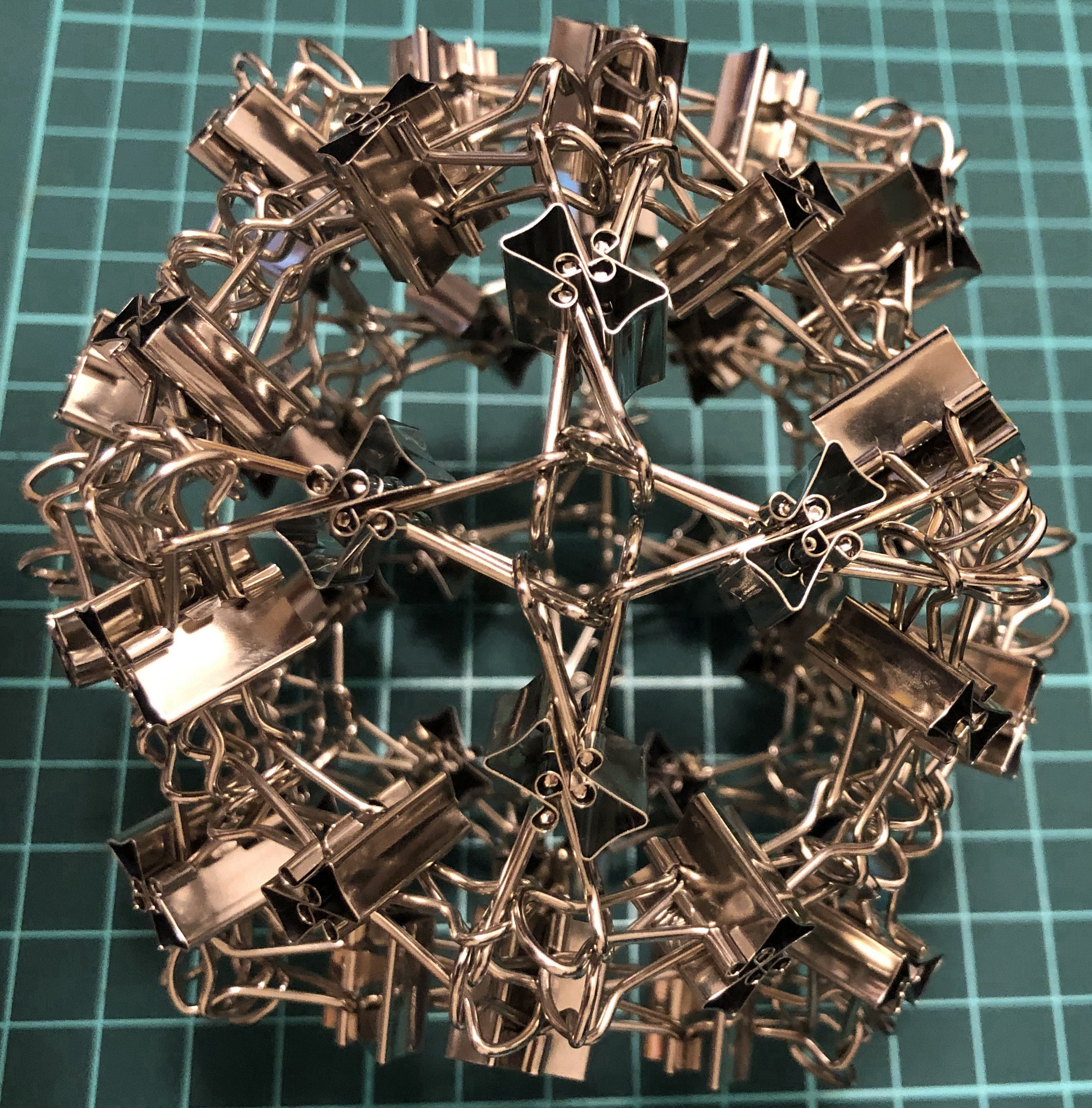
↑ # Clips = 96
↑ Base = deltoidal icositetrahedron
↑ Face config = 3.4.4.4
↑ Symmetry = cube’s rotations
↑ I96-aaC

↑ # Clips = 120
↑ Base = rhombic triacontahedron
↑ Face config = 3.5.3.5
↑ Symmetry = dodecahedron’s rotations
↑ Dual = I120-aD
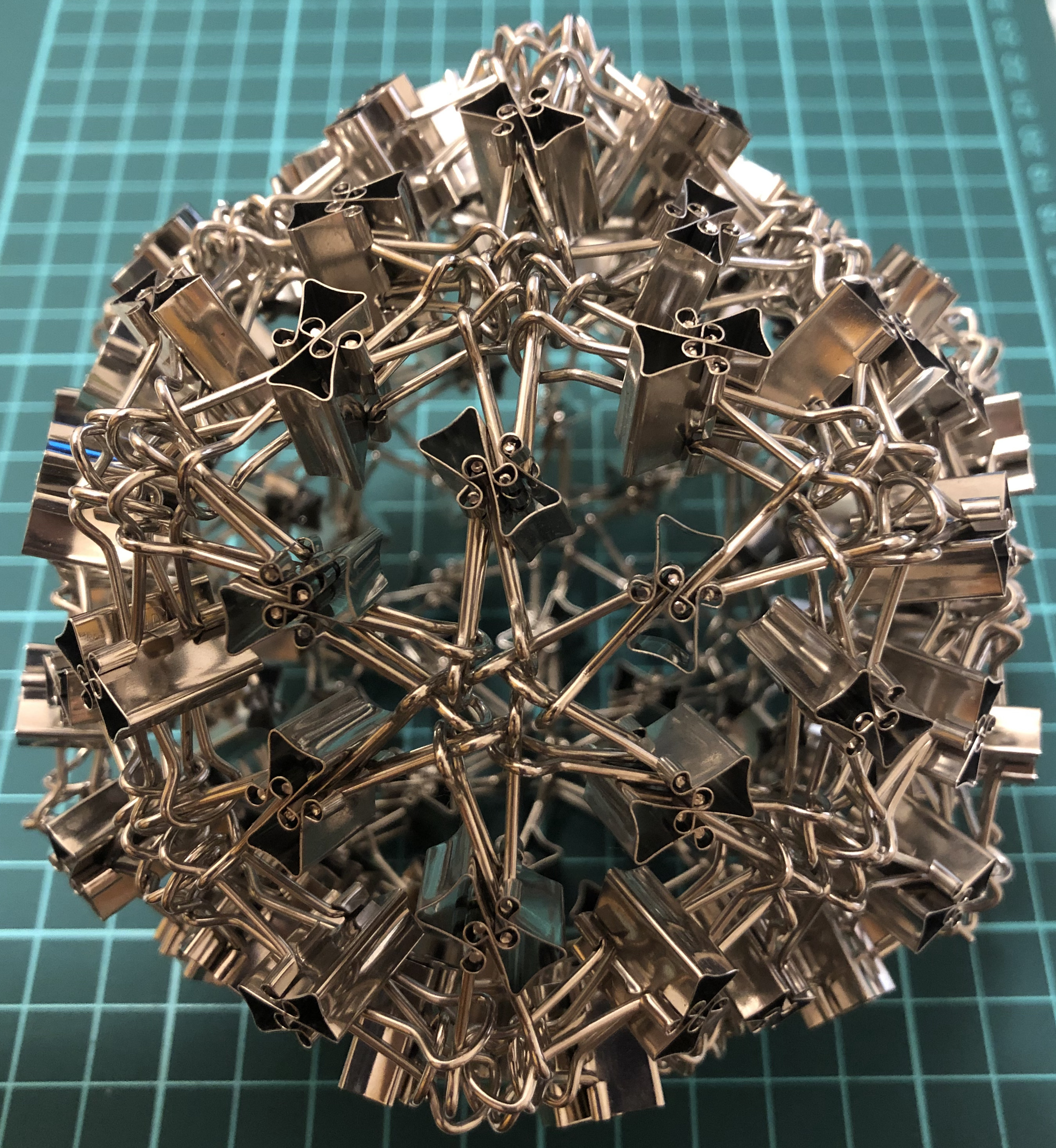
↑ # Clips = 120
↑ Base = pentakis dodecahedron
↑ Face config = 5.6.6
↑ Symmetry = dodecahedron’s rotations
↑ Dual = I180-tI
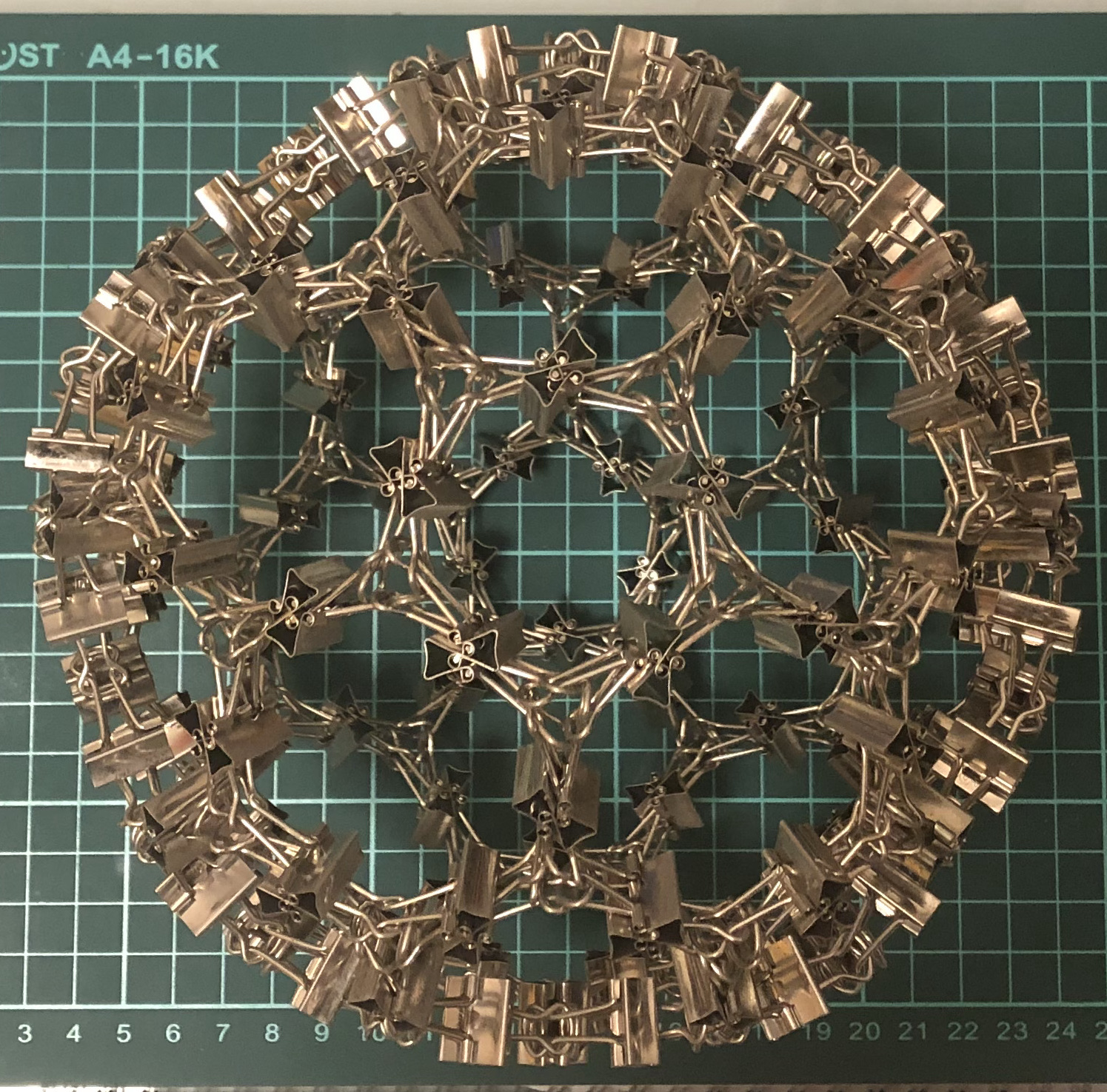
↑ # Clips = 240
↑ Base = chamfered dodecahedron
↑ Each dodecahedron vertex = 4 new vertices
↑ Symmetry = dodecahedron’s rotations
↑ Dual = I240-uI

↑ # Clips = 240
↑ Base = pentakis icosidodecahedron aka C80
↑ Each icosahedron face = 4 small triangles
↑ Symmetry = dodecahedron’s rotations
↑ Dual = I240-cD

↑ # Clips = 12
↑ Base = tetrahedron
↑ Vertex config = 3.3.3
↑ Symmetry = tetrahedron’s rotations
↑ Dual = itself
Difficulty encountered
↑ # Clips = 24
↑ base = cube
↑ Vertex config = 4.4.4
↑ Symmetry = cube’s rotations
↑ dual = W24-O

↑ # Clips = 24
↑ base = octahedron
↑ Vertex config = 3.3.3.3
↑ Symmetry = cube’s rotations
↑ dual = W24-C

↑ # Clips = 48
↑ Base = cuboctahedron
↑ Vertex config = 3.4.3.4
↑ Symmetry = cube’s rotations

↑ # Clips = 60
↑ Base = dodecahedron
↑ Vertex config = 5.5.5
↑ Symmetry = dodecahedron’s rotations
↑ Dual = W60-I

↑ # Clips = 60
↑ Base = icosahedron
↑ Vertex config = 3.3.3.3.3
↑ Symmetry = dodecahedron’s rotations
↑ Dual = W60-D

↑ # Clips = 36
↑ Base = truncated tetrahedron
↑ Vertex config = 3.6.6
↑ Symmetry = tetrahedron’s rotations

↑ # Clips = 120
↑ Base = icosidodecahedron
↑ Vertex config = 3.5.3.5
↑ Symmetry = dodecahedron’s rotations

↑ # Clips = 240
↑ Base = chamfered dodecahedron
↑ Each dodecahedron vertex = 4 new vertices
↑ Symmetry = dodecahedron’s rotations
For a systematic introduction of polyhedra, checkout Platonic solid and Archimedean solid and its dual Catalan solid and the references therein.
For more on symmetry groups, see Polyhedral group and the references therein.
For the naming scheme, see Conway notation and List_of_geodesic_polyhedra_and_Goldberg_polyhedra. Or play with this interactive web app: polyHédronisme. (Refresh the page to get random example!)

Please email me if you have questions (perhaps you want to teach binder clip sculpture in a class) or contributions (when you make something not seen on this page). Once you have made sufficiently many sculptures, you might as well showcase them on a personal website. Notify me so I can put your link below.
Similar works have been published under the names binder clip sculpture and binder clip ball.
http://zacharyabel.com/sculpture/ by Zachary Abel.
https://www.instructables.com/Binder-Clip-Ball/ by 69valentine.
http://blog.andreahawksley.com/tag/binderclips/ by Andrea Hawksley.
https://binderclippolyhedra.com/ by unknown author.
(Domain expired. Link is kept in case the owner buys it back.)
https://momath.org/mathmonday/math-monday-what-to-make-from-binder-clips/ a news article by George Hart.
https://www.instagram.com/rockylau333/ LEGO counterpart by Rocky Lau.
(Old link is https://www.rocky-lau.com/ but connection timeout.)